Vertical Curves

Vertical Curves are nice! I think they are easier to solve than Horizontal Curves because they are essentially parabola equations (which you should be very used to). This is the formula for vertical curves

$$ y(x) = y_{PVC} + G_1x + \frac{1}{2}Rx^2 $$

\(y(x)\) is the elevation y at x horizontal distance into the curve from the start position

\(y_{PVC}\) is the starting elevation at x = 0.

R is referred to as the rate of gradient change:

$$ R = \frac{G_2 – G_1}{L} $$

\(G_1\) and \(G_2\) are the approach grade (or approach tangent) and exit grade. To keep things simple for myself I always express these in decimal percents (e.g. 0.05 instead of 5%). I do this because I like to express my stations as full numbers like 450 feet instead of 4 + 50.

A lot of surveyors and transit engineers do their math the other way. They use the percent value accompanied with the station value (e.g. 5% for 4.5 stations = 5*4.5 = 22.5 feet). This is ok but only works if your stations are at 100 ft. I would just rather convert the stationing to feet and use decimal percent and it is valuable for you to know the difference between the two styles.

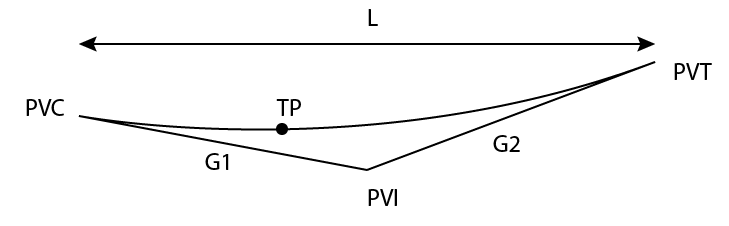
PVC is the beginning of the vertical curve.

PVT is the endpoint of the vertical curve. At this point the grade % takes over OR another vertical curve begins.

PVI is the midpoint between PVC and PVT

TP is the high or low point of the curve. You can find its location (x) with:

$$ x_{TP} = -\frac{G_1}{R}$$

Plug it’s location into the main equation above to find the elevation of the high or low point. Remember that the x from this equation is NOT a station it is a distance from PVC which you may have to convert into the stationing in the problem.

Offset from PVI

If your problem gives you the location of the PVI (middle) and the two gradients you must use a special equation to determine the location of the PVC (beginning) and PVT (end):

$$ \frac{L+2h}{L-2h} = \sqrt{\frac{v-G_1 h}{v-G_2 h}}$$

You must use this because the first equation depends on the fact that PVC is known. x and y in that equation both represent a distance from PVC.