Method of Joints

When analyzing a joint you will solve for the forces in all of the members connected to it.

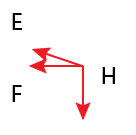
Lets start with H, draw the two unknown forces \(F_{HE}\) and \(F_{HF}\) in tension (away from H).

You already know the downward force is \(5k\). \(F_{HE}\) is the only member with a vertical component so you can solve for it.

$$\sum{F_y} = \frac{10}{\sqrt{1000}}F_{HE} – 5k=0$$

$$ F_{HE} = 5k\frac{\sqrt{1000}}{10}\approx 15.8k (Tension)$$

Ok so you know \(F_{HE}\). Use the horizontal component of \(F_{HE}\) to solve for \(F_{HF}\):

$$\sum{F_x} = -\frac{30}{\sqrt{1000}}F_{HE} – F_{HF} = 0$$

$$ F_{HF} = -\frac{30}{\sqrt{1000}} 15.8k  \approx -15k (Tension) = 15k (Compression) $$

Note: \(\frac{10}{\sqrt{1000}}\) is from the geometry of the structure. \(\sqrt{1000} = \sqrt{10^2 + 30^2}\) and \(\frac{10}{\sqrt{1000}} = \sin\theta\) (where \(\theta\) is the angle of the pointy end).

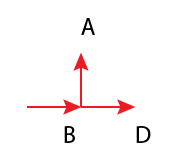
Jumping over to B

You do not know any of these forces. \(F_{BA}\) is a  doozy though. Summing the vertical forces reveals that..

$$ \sum{F_y} = F_{BA} = 0$$

\(F_{BA}\) is zero. You can calculate the horizontal reaction at B by taking moments of the entire truss at A.

$$\sum{M_A} = 10k(10ft) + 10k(20ft) + 10k(30ft) – 10ft(R_{Bx}) = 0$$

$$ R_{Bx} = \dfrac{(100 + 200 + 300)k*ft}{10 ft} =60k$$

Now sum the horizontal forces to find \(F_{BD}\)

$$ \sum{F_x} = 60k + F_{BD} = 0$$

$$ F_{BD} = -60k (Tension) = 60k (Compression)$$

Sliding on over to D

At this joint we know that \(F_{DB} = 60k( Compression)\) (so the arrow should actually point inward for compression), and that the downward force is 5k. We have three unknowns and only two equations (\(\sum{F_x}\) and \(\sum{F_y}\)). This joint is unsolvable so you must move on to another joint before coming back to it.

After practice you start to see the paths to take. At this point E is solvable (secret zero-force member), and then F will be solvable, and then D would be too.

Combining the Method of Joints with the Method of Sections makes things even faster because you can often jump straight to a joint or area that you need for the problem (it may only ask you to find one member for example).